ここでは,3直線で作られる三角形の面積について説明します。
連立方程式を解くことで3直線の3つの交点の座標を求めることができます。
三角形の3つの頂点の座標を求めてしまえば,あとは何とかなるという状況にしましょう。
3直線で作られる三角形の面積を求める方法

ヒロ
3直線で作られる三角形の面積を求める方法を説明する。
三角形の面積を求める方法
- 「2点間の距離の公式」と「点と直線の距離の公式」を利用する。
- 三角形の頂点の座標から,面積公式を利用して面積を求める。
3直線で作られる三角形の面積を求める問題【明治学院大】
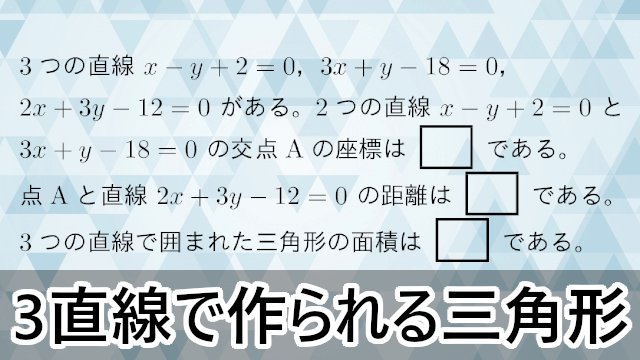
2016年 明治学院大3つの直線 $x-y+2=0$,$3x+y-18=0$,$2x+3y-12=0$ がある。2つの直線 $x-y+2=0$ と $3x+y-18=0$ の交点Aの座標は $\myhako$ である。点Aと直線 $2x+3y-12=0$ の距離は $\myhako$ である。3つの直線で囲まれた三角形の面積は $\myhako$ である。
【考え方と解答】
丁寧に誘導されているため,確実に得点したい問題である。最初の交点Aの座標については,連立方程式を解いて求めよう。
$x-y+2=0~\cdots\cdots①$,$3x+y-18=0~\cdots\cdots②$ とする。$①+②$ より
よって,A$(4,~6)$ である。
点Aと直線 $2x+3y-12=0$ の距離を $d$ とすると
$①\times3+③$ より
$②\times3+③$ より
2点B,Cの座標から線分BCの長さは
丁寧に誘導されているため,確実に得点したい問題である。最初の交点Aの座標については,連立方程式を解いて求めよう。
$x-y+2=0~\cdots\cdots①$,$3x+y-18=0~\cdots\cdots②$ とする。$①+②$ より
\begin{align*}
&4x-16=0 \\[4pt]
&x=4
\end{align*}
①より,$y=6$&4x-16=0 \\[4pt]
&x=4
\end{align*}
よって,A$(4,~6)$ である。
点Aと直線 $2x+3y-12=0$ の距離を $d$ とすると
\begin{align*}
d&=\dfrac{\abs{2\Cdot4+3\Cdot6-12}}{\sqrt{2^2+3^2}} \\[4pt]
&=\dfrac{14}{\sqrt{13}}=\dfrac{14\sqrt{13}}{13}
\end{align*}
$2x+3y-12=0~\cdots\cdots③$ として,2直線①と③の交点をB,②と③の交点をCとする。d&=\dfrac{\abs{2\Cdot4+3\Cdot6-12}}{\sqrt{2^2+3^2}} \\[4pt]
&=\dfrac{14}{\sqrt{13}}=\dfrac{14\sqrt{13}}{13}
\end{align*}
$①\times3+③$ より
\begin{align*}
&5x-6=0 \\[4pt]
&x=\dfrac{6}{5}
\end{align*}
①より&5x-6=0 \\[4pt]
&x=\dfrac{6}{5}
\end{align*}
\begin{align*}
&\dfrac{6}{5}-y+2=0 \\[4pt]
&y=\dfrac{16}{5}
\end{align*}
よって,B$\left(\dfrac{6}{5},~\dfrac{16}{5}\right)$&\dfrac{6}{5}-y+2=0 \\[4pt]
&y=\dfrac{16}{5}
\end{align*}
$②\times3+③$ より
\begin{align*}
&11x-66=0 \\[4pt]
&x=6
\end{align*}
②より&11x-66=0 \\[4pt]
&x=6
\end{align*}
\begin{align*}
&18+y-18=0 \\[4pt]
&y=0
\end{align*}
よって,C$(6,~0)$&18+y-18=0 \\[4pt]
&y=0
\end{align*}
2点B,Cの座標から線分BCの長さは
\begin{align*}
\text{BC}&=\sqrt{\left(\dfrac{6}{5}-6\right)^2+\left(\dfrac{16}{5}-0\right)^2} \\[4pt]
&=\sqrt{\dfrac{8^2(9+4)}{5^2}}=\dfrac{8\sqrt{13}}{5}
\end{align*}
三角形ABCの面積を $S$ とすると\text{BC}&=\sqrt{\left(\dfrac{6}{5}-6\right)^2+\left(\dfrac{16}{5}-0\right)^2} \\[4pt]
&=\sqrt{\dfrac{8^2(9+4)}{5^2}}=\dfrac{8\sqrt{13}}{5}
\end{align*}
\begin{align*}
S&=\dfrac{1}{2}\Cdota\text{BC}\Cdota d \\[4pt]
&=\dfrac{1}{2}\Cdota\dfrac{8\sqrt{13}}{5}\Cdota\dfrac{14}{\sqrt{13}}=\dfrac{56}{5}
\end{align*}
S&=\dfrac{1}{2}\Cdota\text{BC}\Cdota d \\[4pt]
&=\dfrac{1}{2}\Cdota\dfrac{8\sqrt{13}}{5}\Cdota\dfrac{14}{\sqrt{13}}=\dfrac{56}{5}
\end{align*}

ヒロ
三角形の面積だけ求めれば良いのなら,3つの頂点の座標から面積を求めることもできる。
【別の考え方と解答】
上の計算から,3つの頂点の座標はA$(4,~6)$,B$\left(\dfrac{6}{5},~\dfrac{16}{5}\right)$,C$(6,~0)$ と分かる。三角形の面積公式から
上の計算から,3つの頂点の座標はA$(4,~6)$,B$\left(\dfrac{6}{5},~\dfrac{16}{5}\right)$,C$(6,~0)$ と分かる。三角形の面積公式から
\begin{align*}
S&=\dfrac{1}{2}\abs{\left(\dfrac{6}{5}-4\right)\Cdota(0-6)-(6-4)\Cdota\left(\dfrac{16}{5}-6\right)} \\[4pt]
&=\dfrac{1}{2}\abs{\dfrac{84}{5}+\dfrac{28}{5}}=\dfrac{56}{5}
\end{align*}
と求めることができる。S&=\dfrac{1}{2}\abs{\left(\dfrac{6}{5}-4\right)\Cdota(0-6)-(6-4)\Cdota\left(\dfrac{16}{5}-6\right)} \\[4pt]
&=\dfrac{1}{2}\abs{\dfrac{84}{5}+\dfrac{28}{5}}=\dfrac{56}{5}
\end{align*}