ここでは三角比の相互関係について説明します。
三角比の相互関係は直角三角形において成り立つ三平方の定理が元になっています。
三角比の定義と三平方の定理を知っていれば,三角比の相互関係をいつでも導けるようになります。
結果として忘れなくなります。
三角比の相互関係を使いこなせるようにしましょう。
三角比の相互関係

ヒロ
$\sin\theta,~\cos\theta,~\tan\theta$ にはどのような関係があるのかを知ろう。
【三角比の相互関係】
次のような直角三角形ABCを考える。
$a,~b$ をそれぞれ $c$ と $\theta$ で表すと
次のような直角三角形ABCを考える。
$a,~b$ をそれぞれ $c$ と $\theta$ で表すと
\begin{align*}
&a=c\cos\theta \\[4pt]
&b=c\sin\theta
\end{align*}
である。三平方の定理より&a=c\cos\theta \\[4pt]
&b=c\sin\theta
\end{align*}
\begin{align*}
&a^2+b^2=c^2 \\[4pt]
&(c\cos\theta)^2+(c\sin\theta)^2=c^2 \\[4pt]
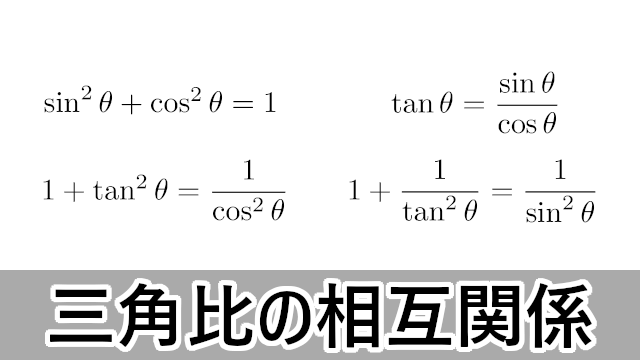
&\cos^2\theta+\sin^2\theta=1
\end{align*}
ABの傾きは $\dfrac{b}{a}$ であり,$\tan\theta$ に等しいから&a^2+b^2=c^2 \\[4pt]
&(c\cos\theta)^2+(c\sin\theta)^2=c^2 \\[4pt]
&\cos^2\theta+\sin^2\theta=1
\end{align*}
\begin{align*}
&\dfrac{c\sin\theta}{c\cos\theta}=\tan\theta \\[4pt]
&\dfrac{\sin\theta}{\cos\theta}=\tan\theta
\end{align*}
$\cos^2\theta+\sin^2\theta=1$ の両辺を $\cos^2\theta$ で割ると&\dfrac{c\sin\theta}{c\cos\theta}=\tan\theta \\[4pt]
&\dfrac{\sin\theta}{\cos\theta}=\tan\theta
\end{align*}
\begin{align*}
&1+\dfrac{\sin^2\theta}{\cos^2\theta}=\dfrac{1}{\cos^2\theta} \\[4pt]
&1+\tan^2\theta=\dfrac{1}{\cos^2\theta}
\end{align*}
となる。ここまでで教科書に載っている3つの相互関係を導くことができた。理系で数学Ⅲも使う場合は,4つ目の相互関係も使いこなせるようにしておくのが良いだろう。$\cos^2\theta+\sin^2\theta=1$ の両辺を $\sin^2\theta$ で割ると&1+\dfrac{\sin^2\theta}{\cos^2\theta}=\dfrac{1}{\cos^2\theta} \\[4pt]
&1+\tan^2\theta=\dfrac{1}{\cos^2\theta}
\end{align*}
\begin{align*}
&\dfrac{\cos^2\theta}{\sin^2\theta}+1=\dfrac{1}{\sin^2\theta} \\[4pt]
&\dfrac{1}{\tan^2\theta}+1=\dfrac{1}{\sin^2\theta}
\end{align*}
となる。覚えていない人が多いけど,覚えておいた方が良い。&\dfrac{\cos^2\theta}{\sin^2\theta}+1=\dfrac{1}{\sin^2\theta} \\[4pt]
&\dfrac{1}{\tan^2\theta}+1=\dfrac{1}{\sin^2\theta}
\end{align*}